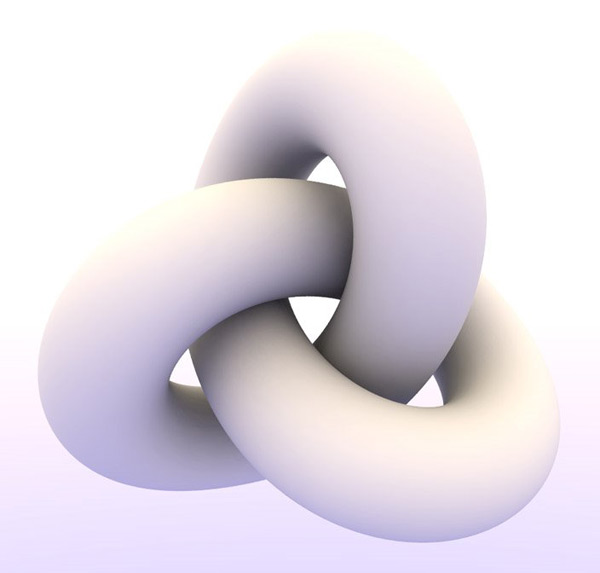
Below is an illustration of the simplest nontrivial knot, the trefoil knot. Knot theory, a subject within the general area of Topology, is the mathematical study of knotting. 

In this study, the tools of mathematics, from algebra to geometry, are applied to answer basic questions about knots. For instance: Given a knot, can it be deformed into a trivial loop? 

The trefoil knot arises in an especially simple way. Consider the equation: 

and the set of solutions: 
Z = \{(x,y) \;|\; x^2 + y^3 = 0 \} \;, 

where x and y are complex numbers. The set Z, a subset of {\mathbb C}^2, is fairly complicated. But the set of vectors (x,y) whose length |x|^2+|y|^2 is equal to one forms the three-dimensional sphere S^3 in {\mathbb C}^2. The intersection of S^3 and Z is the trefoil knot. 

Six of the mathematicians at Indiana University work or have worked in knot theory: Jim Davis, Allan Edmonds, Paul Kirk, Charles Livingston, Kent Orr, and Vladimir Turaev. - Charles Livingston