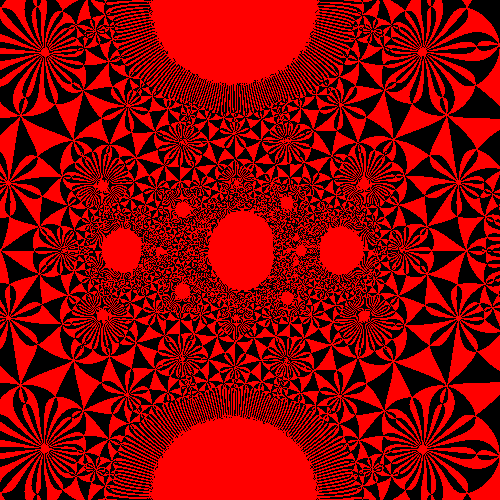
The image below shows preimages of the upper and lower half-planes under several iterates of the rational function 

Thinking of the extended complex plane as two equilateral triangles glued together along their boundaries, with the real axis being the "seam", the preimage of the real axis effects, combinatorially, the barycentric subdivision of each face. Each triangle in the figure is conformally equivalent, in an essentially unique way, to an equilateral triangle, and any two triangles in the figure are related to one another by a series of analytic reflections in a sequence of sides. 

The centers of the large red regions are vertices of bent and very, very skinny triangles: as the number of iterates increases, the number of triangles meeting at the points zero, one, and infinity tends to infinity exponentially fast, while their diameters remain bounded from below.- Kevin M. Pilgrim