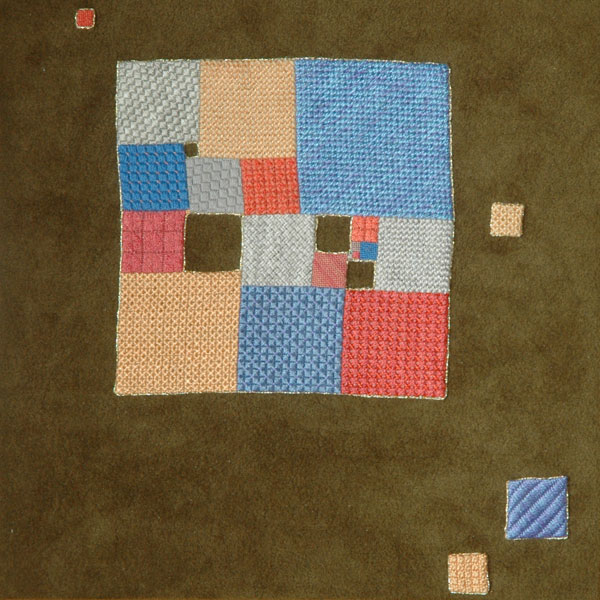
A perfect square is a large square subdivided into smaller squares, where no two of the smaller squares are allowed to be of equal size. It was originally very unclear whether perfect squares existed, but in 1939, R. Sprague found one with 55 smaller squares. This example, with 24 smaller squares (the tiniest, 1 by 1, 2 by 2, and 3 by 3, may be a bit difficult to see) was found by T. H. Willcocks in 1948. Later it turned out that the least number of smaller squares in a perfect square was 21. In this embroidery, four of the squares are still lying around, waiting to be put into their slots---the puzzle is at the stage when it is not yet completely solved, but it is already clear how the solution will go.- Ayelet Lindenstrauss