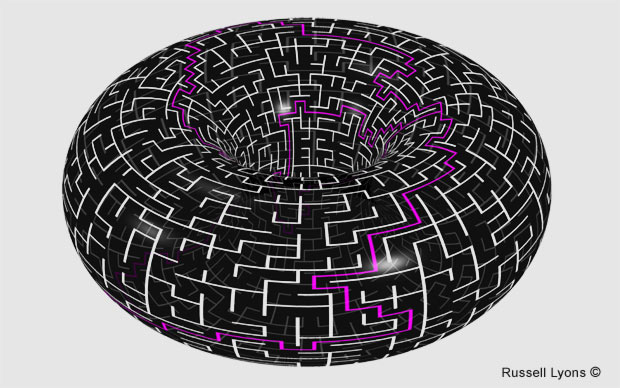
Consider a square grid on a torus. Choose a spanning tree uniformly at random. If we use this spanning tree to form walls, we obtain an interesting maze on the torus. This maze has the homology of a punctured torus, since the walls are contractible to a point. Thus, there must be some paths in the maze that represent some non-contractible cycles. How long are these cycles? There is good reason to think that on an $n \times n$  grid, their length is roughly $n^{5/4}$, but no one knows how to prove this.- Russell Lyons